Introduction
Semiconductor material research and device testing often involve determining the resistivity and Hall mobility of a sample. The resistivity of semiconductor material is primarily dependent on the bulk doping. In a device, the resistivity can affect the capacitance, the series resistance, and the threshold voltage.
The resistivity of the semiconductor is often determined using a four-point probe technique. With a four-probe, or Kelvin, technique, two of the probes are used to source current and the other two probes are used to measure voltage. Using four probes eliminates measurement errors due to the probe resistance, the spreading resistance under each probe, and the contact resistance between each metal probe and the semiconductor material. Because a high impedance voltmeter draws little current, the voltage drops across the probe resistance, spreading resistance, and contact resistance are very small.
Two common Kelvin techniques for determining the resistivity of a semiconductor material are the four-point collinear probe method and the van der Pauw method. The Model 4200-SCS Semiconductor Characterization System can be used for both. Because of its high input impedance (>1016Ω) and accurate low current sourcing, the Model 4200- SCS with preamps is ideal for high resistance samples. This application note explains how to make resistivity measurements of semiconductor materials using the Model 4200-SCS.
The Four-Point Collinear Probe Method
The most common way of measuring the resistivity of a semiconductor material is by using a four-point collinear probe. This technique involves bringing four equally spaced probes in contact with a material of unknown resistance. The probe array is placed in the center of the material, as shown in Figure 1.

The two outer probes are used for sourcing current and the two inner probes are used for measuring the resulting voltage drop across the surface of the sample. The volume resistivity is calculated as follows:

where: ρ = volume resistivity (Ω-cm)
V = the measured voltage (volts)
I = the source current (amperes)
t = the sample thickness (cm)
k* = a correction factor based on the ratio of the probe to wafer diameter and on the ratio of wafer thickness to probe separation
* The correction factors can be found in standard four-point probe resistivity test procedures such as SEMI MF84-02 Test Method for Measuring Resistivity of Silicon Wafers With an In-Line Four-Point Probe.
Using the Model 4200-SCS to Make FourPoint Collinear Probe Measurements
The Model 4200-SCS can make four-point collinear probe measurements using either three or four SMUs (source-measure units). When using three SMUs, all three SMUs are set to Current Bias (voltmeter unit). However, one SMU will source current and the other two will be used to measure the voltage difference between the two inner probes. An example of how this can be set up with the Model 4200-SCS is shown in Figure 2. One SMU (SMU1) and the GNDU (ground unit) are used to source current between the outer two probes. Two other SMUs (SMU2 and SMU3) are used to measure the voltage drop between the two inner probes.

This four-point probe measurement can be set up in the Model 4200-SCS's Keithley Interactive Test Environment (KITE) by using the following steps:
- Begin a new project.
- Create a new sub-site plan.
- Add a new device for a generic or custom-designed four-terminal device.
- Add an ITM (Interactive Test Module) and set up the SMUs as shown in Figure 2. Make sure that the Current Measurement option is checked for SMU1, which is set up as a Current Bias. This current source value will be used to calculate the resistivity. Click the check boxes for the Voltage Measure options for SMU2 and SMU3. Set the source range for SMU2 and SMU3 to 1nA. In general, the current source range determines the input impedance of the SMU as a voltmeter. The lower the current range is, the higher the input impedance will be.
- Change the mode to "Sampling."
- In the ITM Timing menu, choose the number of samples to take. If the Timestamp Enabled option is selected, graphs of voltage vs. time can be plotted and used to determine the settling time.
- In the Formulator menu, type in the formula for the sheet resistivity. First, calculate the voltage difference between SMU2 (BV) and SMU3 (CV). This is VDIFF=BV-CV. For the sheet resistivity (ohms/square), RESISTIVITY=4.532*(VDIFF/AI). To determine the volume resistivity, multiply the sheet resistivity by the thickness of the sample in centimeters (cm).
- After connecting the four-point probe to the wafer, run the program by clicking on the green arrow key. The final resistivity value will be shown in the RESISTIVITY column of the data sheet.
Example Application for Making Four-Point Collinear Probe Measurements with the Model 4200-SCS
An example four-point probe resistivity application has already been created for the Model 4200-SCS. This project was developed using the steps described previously. The name of the project is FourPtProbe. A screen capture of the project is shown in Figure 3. A custom-designed device structure is used to illustrate the four-point measurement.
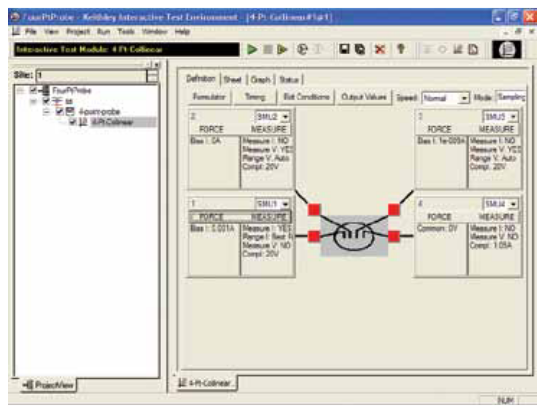
In this project, three SMUs and the GNDU (ground unit) are used to measure the resistivity. For SMU1, enter an appropriate test current, depending on the resistivity of the sample. The green arrow key is used to execute the project. Under the Sheet tab, the resistivity will appear. A correction factor may be applied by using the Formulator menu or by entering a formula on the Calc sheet within the Sheet tab.
van der Pauw Resistivity Measurement Method
The van der Pauw method involves applying a current and measuring voltage using four small contacts on the circumference of a flat, arbitrarily shaped sample of uniform thickness. This method is particularly useful for measuring very small samples because geometric spacing of the contacts is unimportant. Effects due to a sample's size, which is the approximate probe spacing, are irrelevant.
Using this method, the resistivity can be derived from a total of eight measurements that are made around the periphery of the sample with the configurations shown in Figure 4.
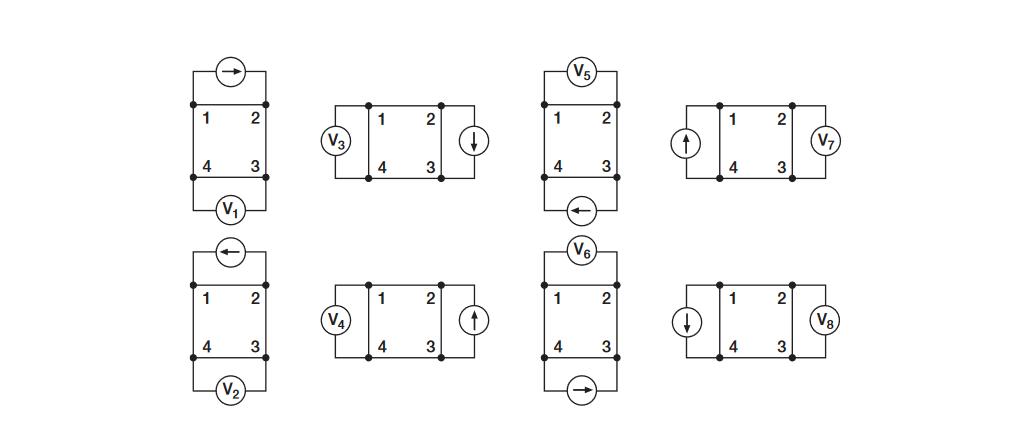
Once all the voltage measurements are taken, two values of resistivity, ρA and ρB, are derived as follows

where: ρA and ρB are volume resistivities in ohm-cm;
ts is the sample thickness in cm;
V1-V8 represents the voltages measured by the voltmeter;
I is the current through the sample in amperes;
fA and fB are geometrical factors based on sample symmetry. They are related to the two resistance ratios QA and QB as shown in the following equations (fA = fB = 1 for perfect symmetry).
QA and QB are calculated using the measured voltages as follows:

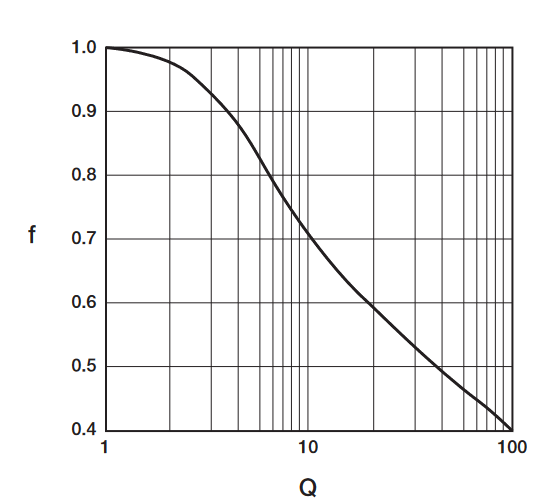
Also, Q and f are related as follows:
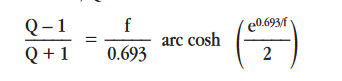
A plot of this function is shown in Figure 5. The value of f can be found from this plot once Q has been calculated.
Once ρA and ρB are known, the average resistivity (ρAVG) can be determined as follows:
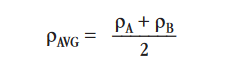
Test Equipment
The electrical measurements for determining van der Pauw resistivity require a current source and a voltmeter. To automate measurements, one might typically use a programmable switch to switch the current source and the voltmeter to all sides of the sample. However, the Model 4200-SCS is more efficient than this.
The Model 4200-SCS with four SMUs and four preamps (for high resistance measurements) is an ideal solution for measuring van der Pauw resistivity, and should enable measurements of resistances greater than 1012W. Since each SMU can be configured as a current source or as a voltmeter, no external switching is required, thus eliminating leakage and offsets errors caused by mechanical switches. This removes the need for additional instruments and programming.
For high resistance materials, a current source that can output very small current with a high output impedance is necessary. A differential electrometer with high input impedance is required to minimize loading effects on the sample. On the lowest current source ranges (1pA and 10pA) of the Model 4200-SCS, the input resistance of the voltmeter is >1016Ω.
Using the Model 4200-SCS to Measure Resistivity with the van der Pauw Method
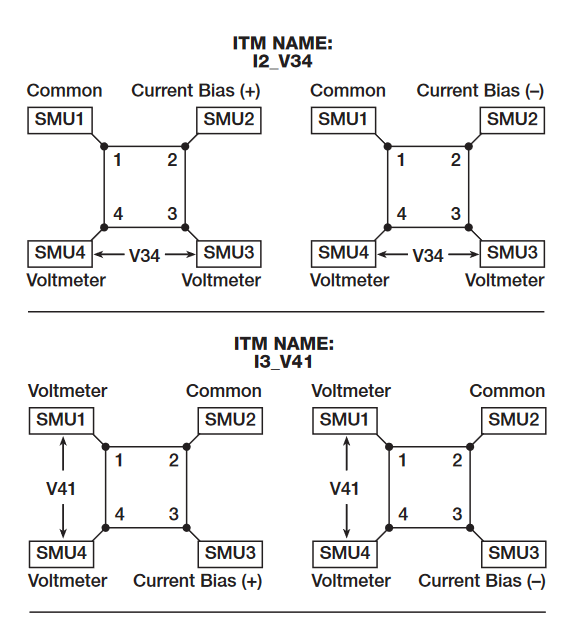
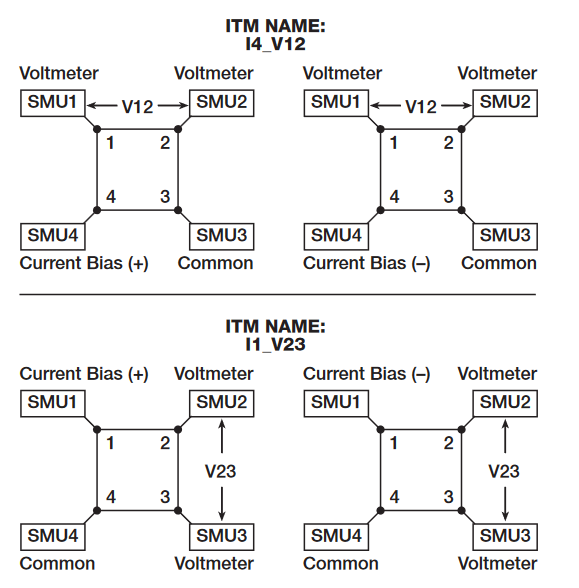
Each terminal of the sample is connected to one SMU, so a Model 4200- SCS with four SMUs is required. A test project with four ITMs (Interactive Test Modules) is written using a generic or custom-designed four-terminal device. Each ITM is a different measurement setup. An example of how the four SMUs are configured in the four ITMs is shown in Figure 6. For each ITM, three of the SMUs are configured as a current bias and a voltmeter (VMU). One of these SMUs applies the test current and the other two SMUs are used as high impedance voltmeters with a test current of zero amps on a low current range (typically 1nA range). The fourth SMU is set to common. The voltage difference must be calculated between the two SMUs set up as high impedance voltmeters. This measurement setup is duplicated around the sample, with each of the four SMUs changing functions in each of the four ITMs.
Basic Procedure for Setting up a New Project for Measuring van der Pauw Resistivity:
- Create a new project.
- Create a new sub-site plan.
- Add a new device plan. It must be either a generic or customdesigned four-terminal device.
- Four ITMs will need to be created. In each ITM, one SMU will be configured as the current source using the current list sweep function, two SMUs will be configured as voltmeters, and one will be configured as a common. Here is an example setup for a high resistance sample:
Terminal A - SMU1: Set to a two-point Current List Sweep. Enter both the positive and negative values of the appropriate source current. Enter the Compliance level and use the Best Fixed source range. Averaging voltage measurements (from SMU2 and SMU3) taken at both a positive and negative test current will correct for voltage offsets in the circuit.
Terminal B - SMU2: Set to Current Bias (VMU) with a test current of 0A. Set the appropriate compliance voltage, and check the Measure Voltage box (VB). Even though 0A will be output, select an appropriate current source range. The input impedance of the voltmeter is directly related to the current source range. The lower the current source range is, the higher the input impedance will be. However, the lower the current source range, the slower the measurement time will be. For most applications, the 1nA range may be used. However, for very high resistance measurements, use a lower current range.
Terminal C - SMU3: Set up the same as Terminal B.
Terminal D - SMU4: Set the Forcing Function to Common.
Use the Formulator to calculate the voltage difference between SMU2 and SMU3 for both the positive and negative test current. This can be done using an equation such as V23DIFF=VB-VC. Take the absolute values of these numbers (from both the positive and negative test current) using the ABS function with an equation such as V23ABS=ABS(V23DIFF). Then average the two voltage difference values using the AVG function with an equation, such as V23=AVG(ABSV23). In the Output Values window, check the average voltage (V23) so that it is sent to the sub-site data sheet, where it will be used in the resistivity calculation. The magnitude of the current source must also be sent to the sub-site data sheet, so click that check box (I1) as well.
Using four ITMs, this same procedure will need to be repeated around all sides of the sample as shown previously in Figure 4. The average voltages from each ITM are then used to calculate the resistivity on the sub-site Calc sheet. A diagram showing how the SMUs are setup in each ITM is shown in Figure 6.
- To calculate the resistivity in the sub-site level, open up the subsite plan. In the Subsite Setup tab, select Cycle Mode and enter "1" in the Number of Cycles field. The Output Values (voltage differences and test current) will appear on the data sheet at the subsite level. The resistivity is calculated on the Calc sheet from the cell references on the Data sheet. The thickness, coefficients, and correction factors are also input on the Calc sheet for the resistivity equation.
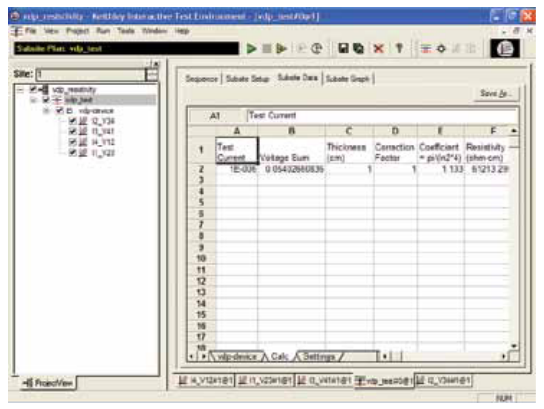
Example van der Pauw Application Using the Model 4200-SCS
An example van der Pauw resistivity project, vdp_resistivity, has been created for KITE 5.0 or higher versions. This project follows the example listed previously. A window of this project is shown in Figure 7.
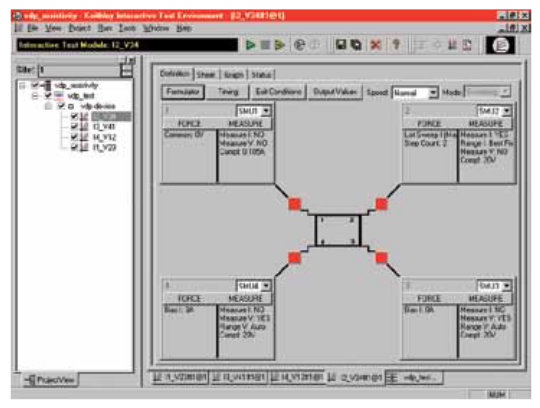
This project has been written following the procedure previously described in this application note. Notice that a van der Pauw device structure is shown in the definition window. The user will need to adjust the source current, the thickness of the material, and the settling time of the measurement.
Adjusting the Source Current
The source current value will need to be modified according to the expected sample resistance. Adjust the current so that the voltage difference will not exceed 25mV (approximately). In each of the four ITMs (I2_V34, I3_V41, I4_V12, I1_V23), enter both polarities of the test current. The same magnitude must be used for each ITM.
Determining the Settling Time
For high resistance samples, it will be necessary to determine the settling time of the measurement. This can be accomplished by sourcing current into two terminals of the sample and measuring the voltage difference between the other two terminals. The settling time can be determined by graphing the voltage difference versus the time of the measurement.
Using the Model 4200-SCS, the voltage versus time graph can be easily created by modifying one of the ITMs described previously. In the Timing menu, take a few hundred or so readings with a sweep time of one second. Make sure that the Timestamp Enabled box is checked. After the readings are done, plot the voltage difference versus time on the graph. (You can choose the parameters to graph by right-clicking on the graph). The settling time is determined from the graph. A timing graph of a very high resistance material is shown in Figure 8.
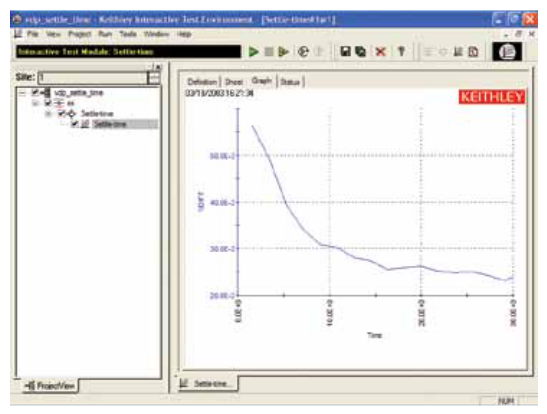
Determine the settling time by visually inspecting the voltage difference vs. time graph. Once the settling time has been determined, use this time as the Sweep Delay (in the Timing menu) for the four resistivity measurement ITMs listed previously. This settling time procedure will need to be repeated for different materials; however, it is not necessary for low resistance materials since they have a short settling time.
Inputting the Thickness of the Sample
The thickness of the material will also need to be entered into the Calc sheet in the sub-site level. Select the sub-site "vdp-test." Go to the Subsite Data tab. It contains the output values of the voltage differences and the test current. From the Calc Sheet tab, the thickness can be adjusted. The default thickness is 1cm. If necessary, a correction factor can also be applied to the resistivity equation.
Running the Project
The "vdp_resistivity" project must be run at the "vdp-test" sub-site level. Make sure that all boxes in the Project/View are checked and highlight "vdp-test." Execute the project by using the sub-site run button (circular arrow). Each time the test is run, the subsite data is updated. The voltage differences from each of the four ITMs (I2_V34, I3_V41, I4_V12, I1_V23) will appear in the Subsite Data "vdp-device" sheet. The resistivity will appear in the Subsite Data "Calc" sheet as shown in Figure 9.
Hall Voltage Measurements
Hall effect measurements are important to semiconductor material characterization because from the Hall voltage, the conductivity type, carrier density, and mobility can be derived. With an applied magnetic field, the Hall voltage can be measured using the configurations shown in Figure 10.
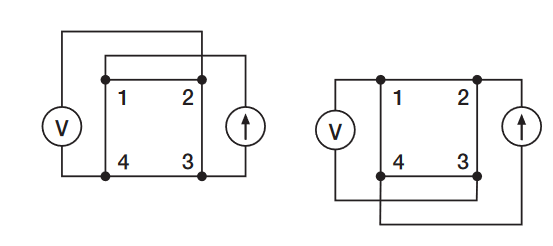
With a positive magnetic field, B, apply a current between terminals 1 and 3, and measure the voltage drop (V2-4+) between terminals 2 and 4. Reverse the current and measure the voltage drop (V4-2+). Next, apply current between terminals 2 and 4, and measure the voltage drop (V1-3+) between terminals 1 and 3. Reverse the current and measure the voltage (V3-1+) again.
Reverse the magnetic field, B, and repeat the procedure again, measuring the four voltages: (V2-4-), (V4-2-), (V1-3-), and (V3-1-).
From the eight Hall voltage measurements, the average Hall coefficient can be calculated as follows:
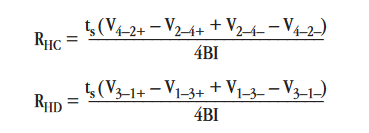
where: RHC and RHD are Hall coefficients in cm3/C;
ts is the sample thickness in cm;
V represents the voltages measured by the voltmeter;
I is the current through the sample in amperes;
B is the magnetic flux in Vs/cm2 (1 Vs/cm2 = 108 gauss)
Once RHC and RHD have been calculated, the average Hall coefficient (RHAVG) can be determined as follows:
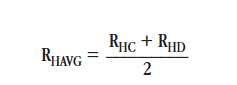
From the resistivity (ρAVG) and the Hall coefficient (RHAVG), the mobility (μH) can be calculated:
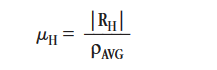
Using the Model 4200-SCS to Measure the Hall Voltage
The setup to measure the Hall voltage is very similar to the setup for measuring resistivity. The difference is the location of the current source and voltmeter terminals. Figure 10 illustrates the setup for Hall voltage measurements. (Figure 4 illustrates the setup for resistivity measurements.) If the user-supplied electromagnet has an IEEE-488 interface, a program can be written in KULT (Keithley User Library Tool) to control the electromagnet using the Model 4200-SCS.
Sources of Error and Measurement Considerations
For successful resistivity measurements, the potential sources of errors need to be considered.
Electrostatic Interference
Electrostatic interference occurs when an electrically charged object is brought near an uncharged object. Usually, the effects of the interference are not noticeable because the charge dissipates rapidly at low resistance levels. However, high resistance materials do not allow the charge to decay quickly and unstable measurements may result. The erroneous readings may be due to either DC or AC electrostatic fields.
To minimize the effects of these fields, an electrostatic shield can be built to enclose the sensitive circuitry. The shield is made from a conductive material and is always connected to the low impedance (FORCE LO) terminal of the SMU.
The cabling in the circuit must also be shielded. Low noise shielded triax cables are supplied with the Model 4200-SCS.
Leakage Current
For high resistance samples, leakage current may degrade measurements. The leakage current is due to the insulation resistance of the cables, probes, and test fixturing. Leakage current may be minimized by using good quality insulators, by reducing humidity, and by using guarding.
A guard is a conductor connected to a low impedance point in the circuit that is nearly at the same potential as the high impedance lead being guarded. The inner shield of the triax connector of the Model 4200-SCS is the guard terminal. This guard should be run from the Model 4200-SCS to as close as possible to the sample. Using triax cabling and fixturing will ensure that the high impedance terminal of the sample is guarded. The guard connection will also reduce measurement time since the cable capacitance will no longer affect the time constant of the measurement.
Light
Currents generated by photoconductive effects can degrade measurements, especially on high resistance samples. To prevent this, the sample should be placed in a dark chamber.
Temperature
Thermoelectric voltages may also affect measurement accuracy. Temperature gradients may result if the sample temperature is not uniform. Thermoelectric voltages may also be generated from sample heating caused by the source current. Heating from the source current will more likely affect low resistance samples, since a higher test current is needed to make the voltage measurements easier. Temperature fluctuations in the laboratory environment may also affect measurements. Since semiconductors have a relatively large temperature coefficient, temperature variations in the laboratory may need to be compensated for by using correction factors.
Carrier Injection
To prevent minority/majority carrier injection from influencing resistivity measurements, the voltage difference between the two voltage sensing terminals should be kept at less than 100mV, ideally 25mV, since the thermal voltage, kt/q, is approximately 26mV. The test current should be kept to as low as possible without affecting the measurement precision.
Alternative Solution
For characterizing low resistance materials, it may be necessary to use a configuration including a programmable current source (such as the Keithley Model 2400), programmable switch matrix (such as the Keithley Model 7001/7012), and the Keithley Model 2182 Nanovoltmeter. The Model 2182 can detect nanovolt-level voltage differences with just 30nV p-p noise (typical).

