오실로스코프란 무엇인가?
오실로스코프(비공식적으로 스코프 또는 오스코프)는 변화하는 신호 전압을 그래픽으로 표시하는 전자 테스트 장비입니다.
Tektronix 오실로스코프의 전체 제품 라인업을 확인하세요. »
오실로스코프 기본 개념
오실로스코프는 다양한 산업 및 분야에서 사용되는 다목적 전자 장비입니다. 엔지니어들은 전자 회로에서 파형 및 신호 분석을 수행하기 위해 오실로스코프를 일반적으로 사용합니다. 회로의 여러 지점에 프로브를 연결함으로써 오실로스코프는 전압 파형을 표시하고 측정하며, 신호 무결성을 분석하고, 회로 문제를 디버깅하며, 신호 주파수와 위상을 분석할 수 있습니다. 실시간으로 전자 신호를 캡처하고 시각화하는 기능을 통해 오실로스코프는 엔지니어들이 회로의 성능을 최적화하고 의도한 대로 작동하는지 확인하는 데 필수적인 도구입니다.
오실로스코프 기본 원리를 이해하는 것은 전자 회로를 효과적으로 진단하고 문제를 해결하는 데 필수입니다.
파형 및 신호 분석
오늘날 거의 모든 소비자 제품은 전자 회로를 포함하고 있습니다. 제품이 단순하든 복잡하든, 전자 부품을 포함하고 있다면 설계, 검증 및 디버깅 과정에서 제품을 작동시키는 수많은 전기 신호를 분석하기 위해 오실로스코프가 필요합니다.
이 간단한 그래프를 통해 신호에 대한 다음과 같은 다양한 정보를 얻을 수 있습니다:
- 신호의 시간 및 전압 값
- 진동하는 신호의 주파수
- 신호로 나타난 회로 내의 ‘동작 부분’
- 신호의 특정 부분이 다른 부분에 비해 얼마나 자주 발생하는지 나타내는 주파수
- 오작동하는 부품이 신호를 왜곡하고 있는지 여부
- 신호에서 직류(DC)와 교류(AC)의 비율
- 신호에서 노이즈가 얼마나 되는지, 그리고 그 노이즈가 시간에 따라 변하는지 등을 파악하는 데 유용
오실로스코프의 그래프
가장 기본적으로 오실로스코프의 그래프는 시간에 따른 신호 전압의 변화를 나타냅니다.
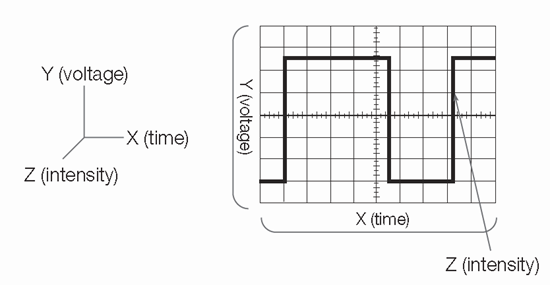
그림 2: 표시된 신호의 X, Y 및 Z 구성 요소
디스플레이의 밝기 또는 강도는 때때로 Z 축으로 불립니다.
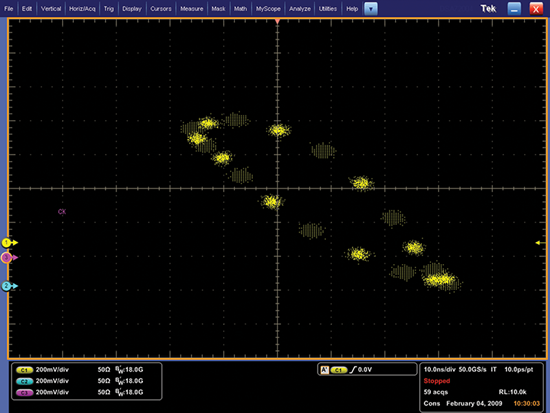
그림 3:Z 축 강도 변조를 가진 두 개의 오프셋 클럭 패턴
신호 무결성의 중요성
오실로스코프의 주요 장점 중 하나는 신호를 정확하게 재구성하는 능력입니다. 신호 재구성이 좋을수록 신호 무결성이 높아집니다. 신호 무결성을 이해하는 한 가지 방법은 다음과 같습니다. 오실로스코프는 신호 이미지를 캡처하여 사용자가 관찰하고 해석하는 카메라와 유사합니다. 신호 무결성의 핵심에는 다음과 같은 몇 가지 주요 문제가 있습니다.
- 사진을 찍을 때, 그것이 실제를 정확하게 나타내는가?
- 사진이 선명한가, 아니면 흐릿한가?
- 초당 얼마나 많은 정확한 사진을 찍을 수 있는가?
오실로스코프의 다양한 시스템과 성능은 신호 무결성을 얼마나 잘 포착하는지 결정합니다.
이 입문서는 이러한 모든 기능을 이해하는 데 도움을 줍니다.
이 정보는 이 장에서 다루며, 파형 및 파형 측정에 대한 기본적인 이해도 제공합니다.
파형과 파형 측정의 이해
시간이 지남에 따라 반복되는 패턴의 일반적인 용어는 파형입니다.
그리고 전압 파형은 모두 반복적인 패턴입니다.
진동, 온도, 전기 신호와 같은 물리적 현상은 파형으로 설명될 수 있습니다.
파형의 형태는 신호에 대한 많은 정보를 나타냅니다. 파형의 높이 변화가 보일 때마다 전압이 변경되었음을 알 수 있습니다. 수평선이 평평하게 이어지는 구간에서는 해당 시간 동안 변화가 없음을 알 수 있습니다.
직선의 대각선은 선형적 변화를 의미하고, 곡선은 비선형적 변화를 나타냅니다.
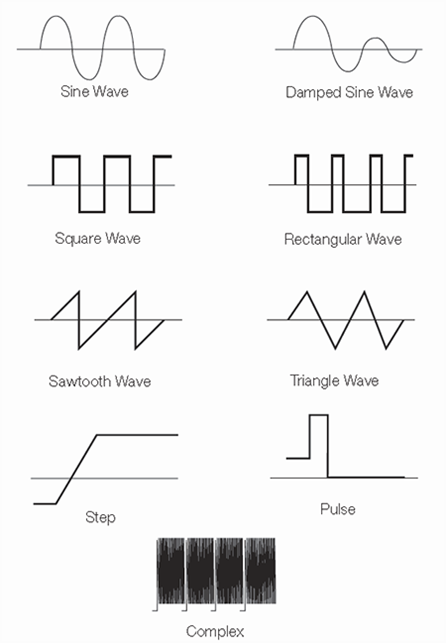
그림 4:일반적인 파형
그림 5는 사인파, 구형파, 삼각파, 톱니파 등 일반적인 파형의 출처를 나타냅니다.
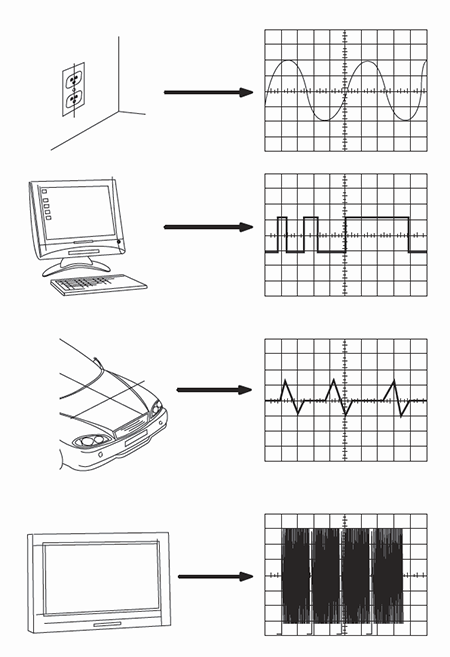
그림 5: 일반적인 파형의 출처
파형의 종류
대부분의 파형을 다음 유형으로 분류할 수 있습니다:
- 사인파
- 구형파 및 직사각형파
- 톱니파 및 삼각파
- 스텝 및 펄스 형태
- 주기적 및 비주기적 신호
- 동기 및 비동기 신호
- 복합 파형
다음으로 각 파형 유형을 자세히 살펴보겠습니다.
사인파
사인파는 여러 가지 이유로 가장 기본적인 파형입니다. 삼각법 수업에서 배웠을 바로 그 사인 함수와 동일한 형태를 가지며, 조화로운 수학적 속성을 지닙니다.
벽면 콘센트의 전압은 사인파 형태로 변동합니다. 신호 발생기의 발진 회로에서 생성되는 테스트 신호도 종종 사인파입니다.
대부분의 교류(AC) 전원은 사인파 형태를 생성합니다. (AC는 교류를 의미하지만, 전압도 교대로 변합니다. DC는 직류를 의미하며, 배터리가 생성하는 것과 같이 전류와 전압이 일정합니다.) 감쇠된 사인파는 발진하지만 시간이 지남에 따라 진폭이 줄어드는 회로에서 볼 수 있는 특별한 경우입니다.
구형파 및 직사각형파
구형파는 또 다른 일반적인 일반적인 파형입니다. 기본적으로 구형파는 일정한 간격으로 켜졌다 꺼지거나 (높은 전압과 낮은 전압 사이를 왔다 갔다 하는) 전압입니다. 이는 증폭기를 테스트하는 데 사용되는 표준 파형입니다. 좋은 증폭기는 구형파의 진폭을 왜곡을 최소화하면서 증가시킵니다.
텔레비전, 라디오 및 컴퓨터 회로는 흔히 구형파를 타이밍 신호로 사용합니다. 구형파와 유사하지만 높은 전압과 낮은 전압이 유지되는 시간 간격이 같지 않은 파형을 구형파라고 합니다. 이는 디지털 회로를 분석할 때 특히 중요합니다.
톱니파 및 삼각파
톱니파와 삼각파는 아날로그 오실로스코프의 수평 스위프나 텔레비전의 래스터 스캔과 같이 전압을 선형적으로 제어하도록 설계된 회로에서 발생합니다.
이러한 파형의 전압 수준 간 전환은 일정한 속도로 변화합니다.
스텝 및 펄스 형태
드물게 발생하거나 비주기적으로 발생하는 스텝 및 펄스와 같은 신호는 과도적(transient) 또는 단발(single-shot) 이벤트로 불립니다.
스텝은 전압이 갑자기 변하는 것을 나타내며, 전원 스위치를 켜는 것과 유사합니다.
펄스는 전압이 갑자기 변화하는 것을 나타내며, 전원 스위치를 빠르게 켰다가 다시 끄는 것과 유사합니다. 펄스는 컴퓨터 회로를 통해 이동하는 1비트의 정보를 나타낼 수도 있고, 회로의 결함이나 이상을 나타낼 수도 있습니다.
함께 이동하는 펄스들의 집합을 펄스 트레인이라고 합니다. 컴퓨터의 디지털 부품들은 펄스를 사용하여 서로 통신합니다. 이러한 펄스는 직렬 데이터 스트림 형태일 수도 있고, 병렬 데이터 버스에서 값을 나타내기 위해 여러 신호선이 사용될 수도 있습니다. 펄스는 엑스레이, 레이더 및 통신 장비에서도 흔히 사용됩니다.
주기적 및 비주기적 신호
반복되는 신호는 주기적 신호라 하고, 반복되지 않는 신호는 비주기적 신호라 합니다.
동기 및 비동기 신호
두 신호 간에 타이밍 관계가 존재하면 동기 신호라고 합니다. 컴퓨터 내부의 클록, 데이터 및 주소 신호는 동기 신호의 예입니다.
비동기 신호는 두 신호 간에 타이밍 관계가 존재하지 않는 신호입니다. 컴퓨터 키보드의 키를 누르는 행위와 컴퓨터 내부의 클록 사이에는 시간적 상관관계가 없기 때문에 이러한 신호는 비동기 신호로 간주됩니다.
복합 파형
일부 파형은 사인파, 구형파, 스텝, 펄스와 같은 다양한 파형 유형의 특성을 결합하여 복잡한 파형을 생성합니다. 신호 정보는 진폭, 위상 및/또는 주파수 변화의 형태로 내장될 수 있습니다.
예를 들어, 그림 6의 신호는 평범한 복합 비디오 신호처럼 보이지만, 실제로는 저주파 엔벨로프 내에 임베딩된 다수의 고주파 파형 사이클로 구성되어 있습니다.
이 예시에서는 스텝들의 상대적인 레벨과 타이밍 관계를 이해하는 것이 중요합니다. 이 신호를 보려면 저주파 엔벨로프를 캡처하고 고주파 파형을 강도별로 혼합하여 전체적인 조합을 시각적으로 해석할 수 있는 이미지로 표시하는 오실로스코프가 필요합니다.
디지털 포스퍼 오실로스코프(DPO)는 그림 6에 표시된 비디오 신호와 같은 복잡한 파형을 보는 데 가장 적합합니다. 이 장비의 디스플레이는 파형이 실제로 어떻게 동작하는지 이해하는 데 필수적인 빈도 발생 정보 또는 강도 등급을 제공합니다.
일부 오실로스코프는 특정 복합 신호를 분석하기 위한 디코딩 기능을 갖추고 있습니다.
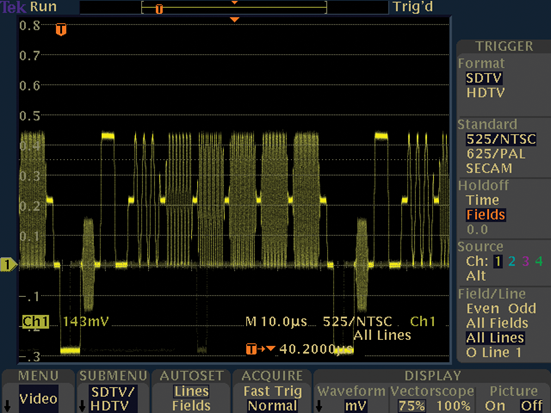
그림 6: NTSC 복합 영상 신호는 복잡한 파형의 한 예시임
통신 분야의 디지털 데이터 신호는 복잡한 패턴과 높은 주파수 대역폭을 가질 수 있습니다.
아이 패턴은 리시버에서 들어오는 디지털 데이터를 샘플링하여 수직축에 적용하고, 데이터 전송률을 이용하여 수평 스위프를 트리거할 때 생성됩니다. 아이 패턴은 가능한 모든 에지 변화와 상태가 중첩된 하나의 포괄적인 뷰로 데이터의 1비트 또는 단위 간격을 표시합니다.
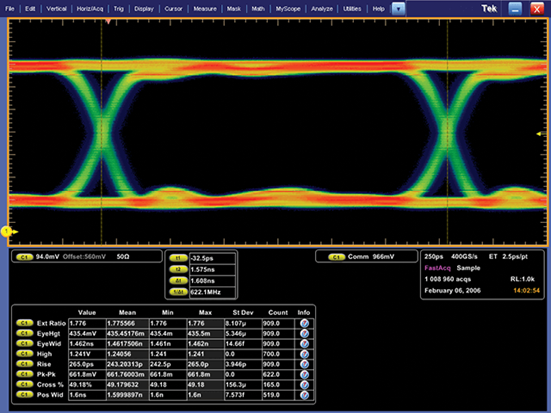
그림 7: 622Mb/s 직렬 데이터의 아이 패턴(Eye Pattern)
별자리 다이어그램(Constellation Diagram)은 디지털 변조 상태를 시각적으로 나타낸 것입니다.

Constellation Diagram (별자리 다이어그램)
파형 측정
파형 측정 유형을 설명하는 데 여러 용어가 사용되며, 이를 명확히 이해하는 것이 중요합니다.
주파수와 주기
신호가 반복되면 주파수를 가집니다. 주파수는 신호가 초당 몇 번 반복되는지를 나타냅니다.
반복 신호는 주기를 가지며, 주기는 신호가 한 번 반복하는 데 걸리는 시간을 의미합니다.
주기와 주파수는 서로 역수 관계에 있습니다.
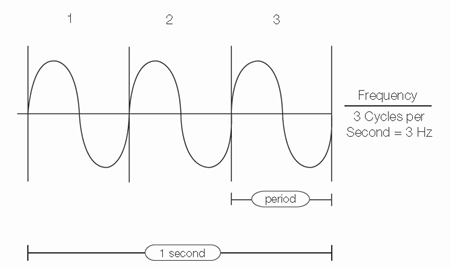
그림 8: 사인파의 주파수와 주기
전압
전압은 회로 내 두 지점 사이의 전기적 퍼텐셜, 즉 신호의 세기를 나타냅니다. 일반적으로 이 두 지점 중 하나는 접지, 즉 0볼트이지만 항상 그런 것은 아닙니다. 파형의 최대 피크에서 최소 피크까지의 전압을 측정할 수도 있으며, 이를 피크-대-피크 전압이라고 합니다.
진폭
진폭은 회로 내 두 지점 사이의 전압 크기를 의미하며, 일반적으로 접지 또는 0볼트 기준점에서 신호의 최대 전압까지를 나타냅니다. 그림 9에 표시된 파형은 진폭이 1V이고 피크-대-피크 전압이 2V입니다.
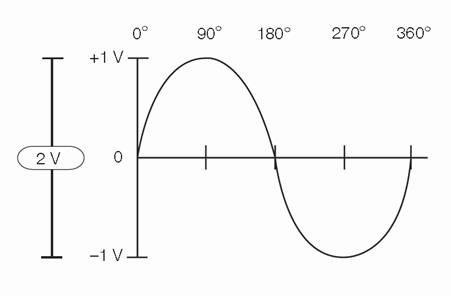
그림 9: 사인파의 진폭과 각도
위상
위상은 사인파를 통해 가장 잘 설명됩니다. 사인파의 전압 레벨은 원 운동을 기반으로 합니다. 원이 360°를 가지므로, 그림 10에서 볼 수 있듯이 사인파의 한 주기는 360°를 가집니다. 기준점으로부터 신호가 수평으로 얼마나 이동했는지를 각도로 나타내는 것이 위상입니다.
각도를 사용하여 파형 주기 중 어느 지점에서든 위상 각도를 나타낼 수 있습니다.
위상 이동은 주기적으로 동일한 두 신호 간의 타이밍 차이를 설명합니다. 그림 10에서 "전압"으로 표시된 파형에 비해 "전류"로 표시된 파형은 90° 위상차가 있다고 합니다. 이는 두 파형이 주기의 1/4만큼 떨어진 지점에서 유사한 점에 도달하기 때문입니다(360°/4 = 90°). 위상 이동은 전자 공학에서 흔히 발생합니다.
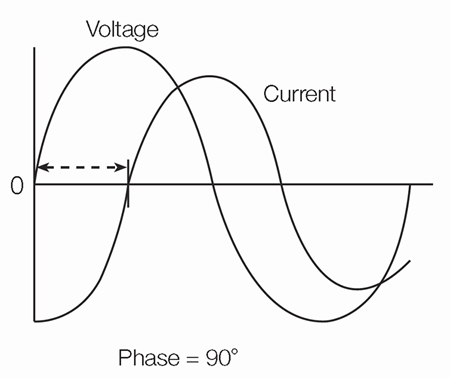
그림 10: 위상 이동
디지털 오실로스코프를 이용한 파형 측정
디지털 오실로스코프는 파형 측정을 쉽고 정확하며 효율적으로 만드는 기능을 갖추고 있습니다. 전면 패널 버튼과 화면 기반 메뉴를 통해 완전히 자동화된 측정을 선택할 수 있습니다. 여기에는 진폭, 주기, 상승/하강 시간 등이 포함됩니다.
많은 디지털 오실로스코프는 평균값(mean)과 실효값(RMS)을 자동으로 측정하여 제공합니다. 또한 듀티 사이클과 기타 수학 연산 기능도 제공합니다. 자동 측정 결과는 화면에 숫자와 문자로 표시됩니다. 일반적으로 이러한 측정값은 눈으로 직접 파형을 읽어 측정하는 것보다 훨씬 정확합니다.