Introduction
Découvrez comment une sonde d'oscilloscope modifie le signal que vous mesurez au point de test et quelles spécifications rechercher pour réduire au maximum ses effets. Un piège de mesure que les utilisateurs oublient souvent ou négligent est l'effet de la charge de la sonde. En fin de compte, vous souhaitez sélectionner une sonde d'oscilloscope qui réponde aux critères suivants :
- Transmettre fidèlement le signal de l'appareil testé à l'oscilloscope
- Ne pas déranger ! ce qui signifie que vous voulez choisir une sonde qui modifie le moins possible le signal au point de test
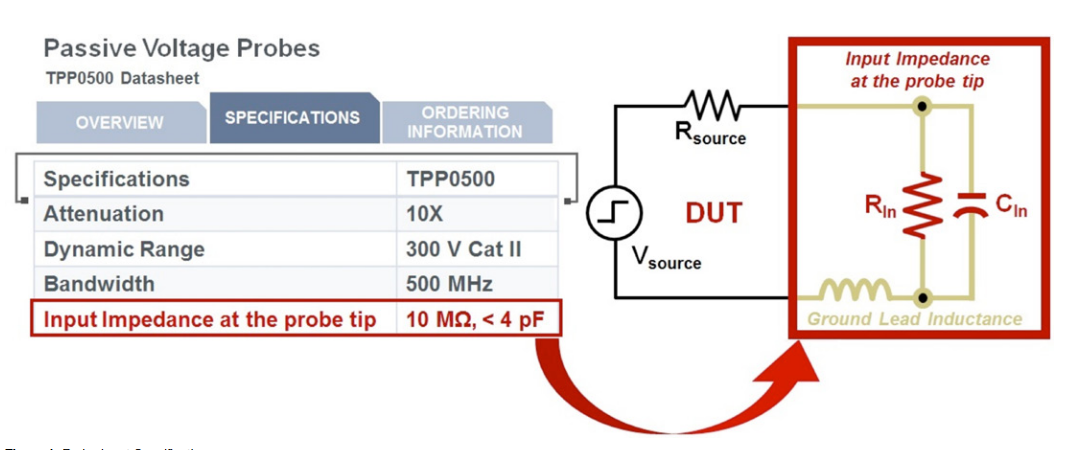
Si je soudais à la masse un condensateur de 15 pF situé à un endroit quelconque de votre circuit, vous attendriez-vous à ce que le circuit se comporte différemment ? Bien sûr que oui. Comme une sonde d'oscilloscope possède une capacité, une résistance et une inductance, elle va affecter le signal que vous mesurez et peut affecter le fonctionnement du circuit. Pour produire une forme d'onde de tension sur l'oscilloscope, la pointe de la sonde va absorber du courant ; elle est conductrice. Comme la pointe de la sonde doit tirer un certain courant, elle va perturber ou charger le circuit. Il existe deux spécifications de sonde liées à la charge de la sonde dans le circuit, et ces facteurs de charge sont la résistance d'entrée et la capacité d'entrée. La figure 1 donne un exemple des spécifications de la fiche technique de l'impédance d'entrée de la sonde et un exemple de la façon dont ces spécifications peuvent être modélisées.
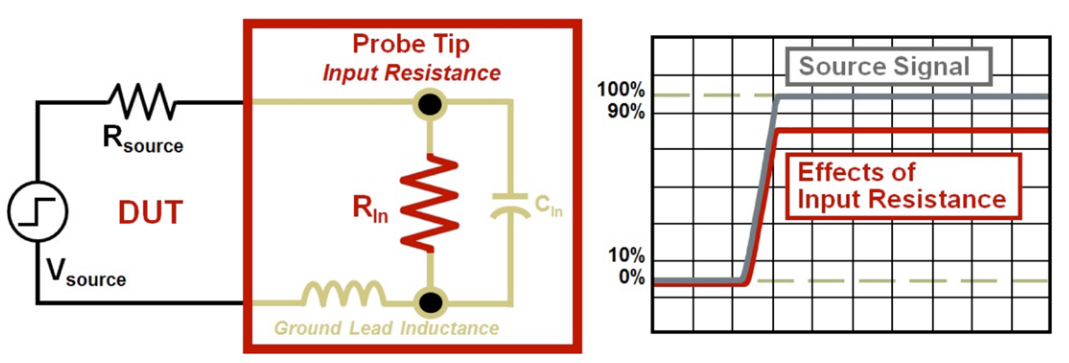
Spécification de la résistance d'entrée
Examinons d'abord la spécification de la résistance d'entrée. La résistance d'entrée de la sonde et de l'oscilloscope réduit l'amplitude du signal testé, comme le montre la forme d'onde à droite de la figure 2. La forme d'onde du signal chargé ressemblera beaucoup au signal source, mais l'amplitude changera. La réduction de l'amplitude du signal source dépend généralement de la résistance de la source, car la plupart des sondes passives livrées avec un oscilloscope ont une très grande résistance d'entrée de 10 MΩ.
La résistance d'entrée de la sonde combinée à la résistance d'entrée de l'oscilloscope fait que le système de mesure agit comme un diviseur de tension. Le calcul de l'effet de la résistance d'entrée est présenté dans l'équation 1.

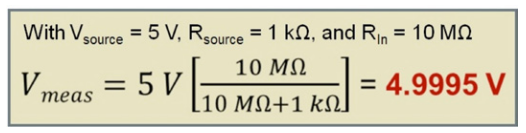
Idéalement, Vmeas devrait être égal àVsource car le signal au point de test serait inchangé. En réalité, la résistance d'entrée de la sonde et de l'oscilloscope aura un impact sur l'amplitude mesurée. Pour minimiser la charge résistive, les sondes ont généralement une grande résistance d'entrée, et pour la plupart des applications, la résistance d'entrée d'une sonde est moins susceptible d'entraîner une charge significative de la sonde puisque la plupart des sondes passives 10X livrées avec un oscilloscope ont une résistance d'entrée de 10 MΩ. L'équation 2 montre comment la grande résistance d'entrée d'un système entraîne une très faible charge ou perturbation du circuit.
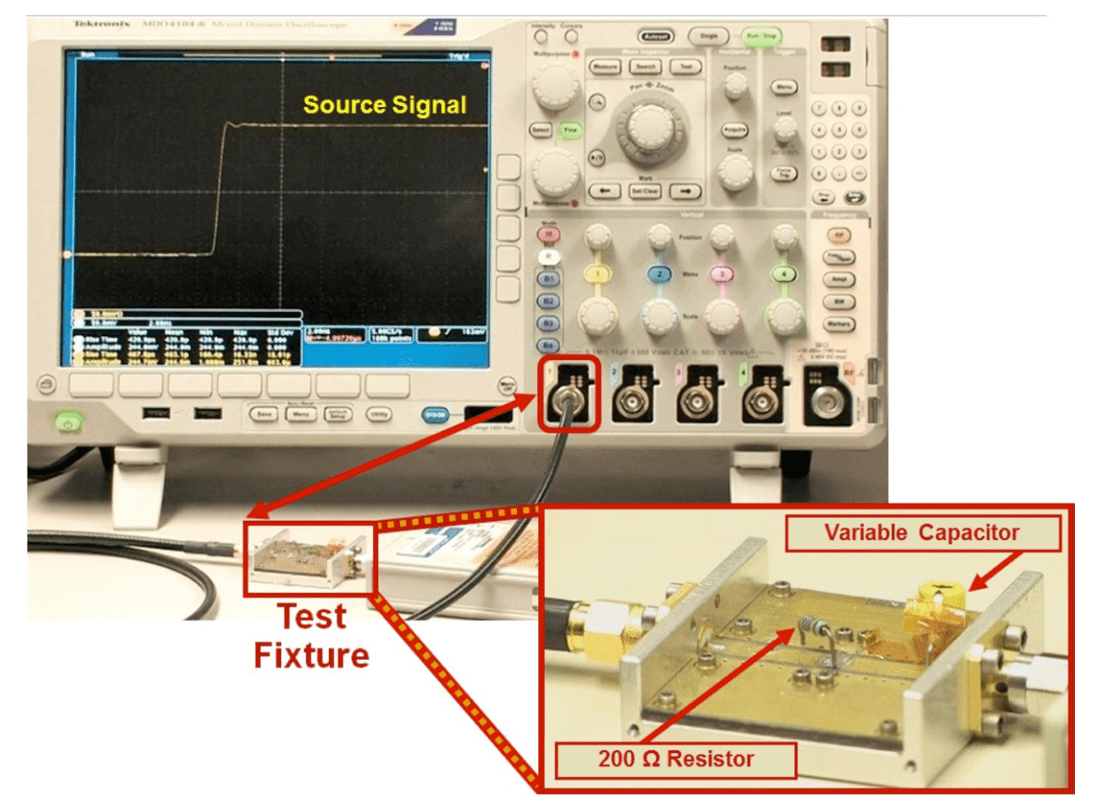
Comme le montre l'équation 2, la résistance d'entrée de 10 MΩ d'un système de mesure a peu d'impact sur le signal mesuré. Dans cet exemple, le signal mesuré est calculé à 4,9995 V, ce qui correspond à 99,99 % du signal source de 5 V. L'examen des équations et des modèles permet d'obtenir une vue d'ensemble théorique de la résistance d'entrée, mais la meilleure méthode pour voir l'impact de la charge de la sonde est de montrer comment le signal au point de test change. La figure 3 présente un montage de test utilisant une source de tension avec une résistance de source de 50 Ω, un montage de test et un oscilloscope. Une résistance de 200 Ω et un condensateur variable sont soudés sur le dispositif de test. Vous pouvez clairement voir que le dispositif de test est directement relié à l'oscilloscope et que la sortie du dispositif de test est connectée à la voie 1. Cette configuration a pour but de montrer ce qui se passe au point de test et d'observer les effets de la charge de la sonde. L'oscilloscope affiche la sortie du dispositif de test, et non la sortie d'une sonde.
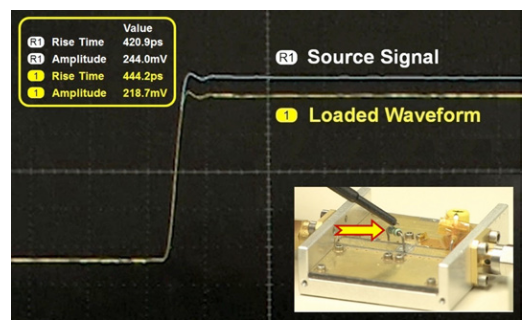
Avec 10 MΩ, il serait difficile de voir l'effet de la résistance d'entrée au niveau de l'objet sous test. Pour voir comment la résistance d'entrée affecte le signal au point de test, la figure 4 montre les effets de la résistance de 200 Ω soudée dans le dispositif de test pour exagérer l'effet. La forme d'onde de référence est le signal source, et le canal 1 est le signal source chargé par la résistance d'entrée de 200 Ω. Les mesures sont activées pour observer l'amplitude et le temps de montée de la forme d'onde de référence et de la forme d'onde chargée. La forme de l'onde chargée est similaire au signal source, tout comme le temps de montée, mais l'amplitude a été réduite.
Spécification de la capacité d'entrée
Lorsqu'ils choisissent la bonne sonde pour leur application, la plupart des utilisateurs s'intéressent à la bande passante, à la plage dynamique et peut-être même à la spécification de la résistance d'entrée de la sonde. Lorsqu'ils évaluent les caractéristiques de charge d'une sonde, la plupart des utilisateurs voient la valeur de la résistance d'entrée de 10 MΩ et en concluent que la sonde a des capacités de charge suffisantes. La capacité d'entrée de la sonde est une spécification critique presque toujours négligée. Lors de la mesure de signaux à basses fréquences, la capacité de la sonde a une réactance très élevée et la charge de la sonde n'est pas aussi importante. Cependant, lorsque la fréquence du signal mesuré augmente, la réactance capacitive diminue. À des fréquences plus élevées, l'impédance de la sonde diminue, ce qui entraîne une charge beaucoup plus importante de la sonde. Plus la capacité d'entrée de la sonde est grande, plus elle va perturber ou charger le circuit lorsque la fréquence mesurée augmente.
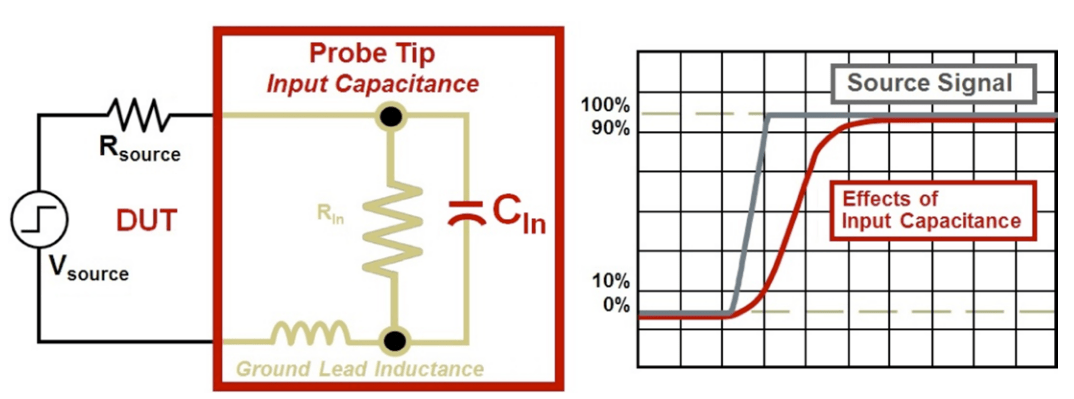
L'effet de la capacité d'entrée est illustré sur la forme d'onde à droite de la figure 5. Par rapport au signal source, la forme d'onde affectée par une valeur de capacité d'entrée plus élevée présente un coin avant arrondi. La conséquence d'une grande valeur de capacité est grave car le bord avant du signal contient les hautes fréquences. Lorsque ce contenu critique du signal a été compromis, on peut se demander si les résultats de la mesure sont fiables. Lors du choix d'une sonde, les utilisateurs doivent identifier une solution de palpage avec la capacité d'entrée la plus faible.

En utilisant le modèle simple de la figure 5, une équation de premier ordre montre qu'une capacité d'entrée plus importante se traduira par des temps de montée beaucoup plus lents et une bande passante plus faible. Sur la base du calcul du temps de montée dans l'équation 3, il existe une relation évidente entre une capacité d'entrée plus importante et des temps de montée plus lents.
En utilisant la configuration de test de la figure 3, le condensateur variable soudé dans le dispositif de test sera maintenant connecté à la ligne de bande de signal pour montrer les effets de la capacité d'entrée de la sonde. Avec le condensateur variable réglé à 50 pF, l'impact de la charge capacitive est illustré à la figure 6. Là encore, le signal source est représenté par la forme d'onde de référence identifiée par R1 et le canal 1 est la sortie du dispositif de test, c'est-à-dire la forme d'onde chargée par le condensateur variable.
Les mesures révèlent les différences d'amplitude et d'élévation entre la forme d'onde de référence et la forme d'onde chargée. Le bord avant a été dégradé, le temps de montée est nettement plus lent et il y a un léger déphasage.
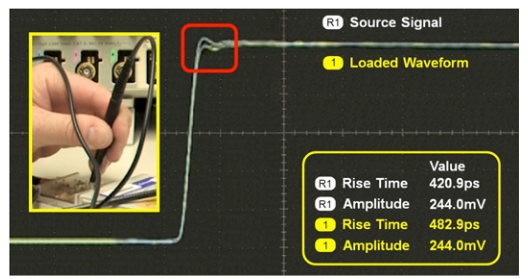
Comment la sonde passive livrée avec votre oscilloscope charge-t-elle votre signal ?
Le dispositif d'essai des sections précédentes utilisait des composants résistifs et capacitifs simples pour isoler les effets de la résistance et de la capacité d'entrée. Intéressons-nous maintenant à l'impact de la capacité d'entrée induite par certaines sondes passives livrées avec un oscilloscope.
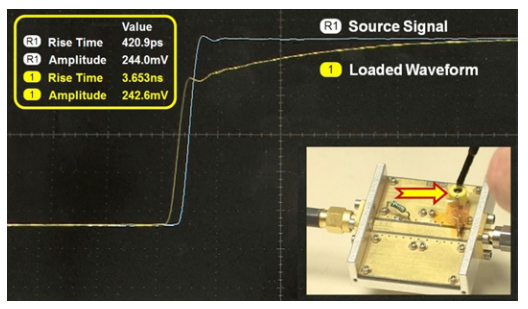
Examinons d'abord les effets de charge d'une sonde passive d'Agilent, livrée en standard avec l'oscilloscope. Cette sonde présente de nombreuses caractéristiques recherchées par les utilisateurs : Largeur de bande de 500 MHz, plage dynamique de 300 V CAT II et résistance d'entrée de 10 MΩ. Cette sonde a également une capacité d'entrée de 9,5 pF, ce qui est comparable aux sondes passives proposées par d'autres fournisseurs. En utilisant la même configuration de test que celle de la figure 3, la sonde passive Agilent sera connectée au montage de test comme indiqué dans le coin supérieur gauche de la figure 7. La pointe de la sonde est appliquée sur le trajet du signal et un court ressort de masse est connecté à la terre. L'impact de la capacité d'entrée est évident. Le coin avant de la forme d'onde chargée de la figure 7 est dégradé et le temps de montée est nettement plus lent. Le signal que vous mesurez maintenant ressemble très peu au signal source original. Ce signal dégradé est encore modifié lorsqu'il passe par le câble de la sonde jusqu'au boîtier de compensation de la sonde et enfin jusqu'à l'oscilloscope. Ce signal de test doit être reconstruit par la sonde et l'oscilloscope pour qu'il ressemble au signal original. Ce type de charge ou de perturbation aura des effets néfastes lors de la validation de circuits importants.
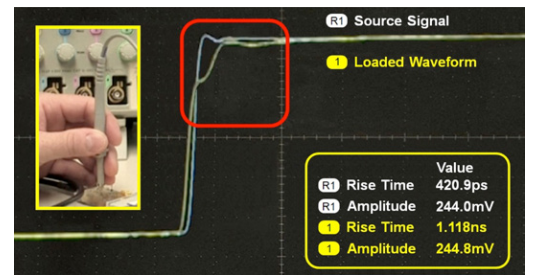
En raison des effets négatifs de la charge capacitive, Tektronix inclut une nouvelle série de sondes passives dans ses oscilloscopes avec une capacité d'entrée de 3,9 pF, la meilleure de l'industrie. Ces produits sont le TPP1000, le TPP0500B et le TPP0250. En connectant un TPP0500B au montage de test comme indiqué dans la figure 8, les avantages d'une sonde à faible capacité d'entrée sont évidents. Le signal sur le canal 1, qui est la forme d'onde chargée, est presque identique au signal source. En outre, le temps de montée de la forme d'onde chargée est très peu affecté. Une fois de plus, vous souhaitez effectuer des mesures avec une sonde qui sera la moins nuisible possible, c'est-à-dire qui n'affectera que très peu le signal que vous mesurez. Une sonde passive à faible capacité de Tektronix offre pratiquement les mêmes avantages qu'une sonde active en termes de charge de la sonde.
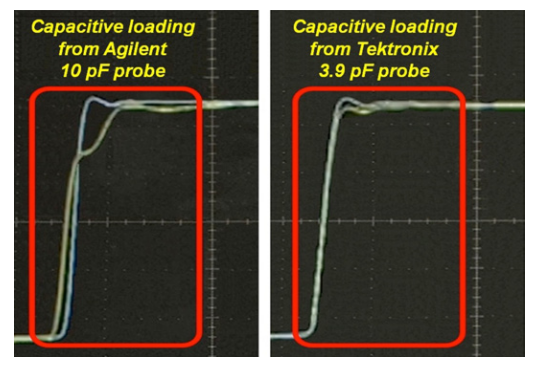
La figure 9 compare la charge capacitive d'une sonde standard Agilent à celle de la sonde TPP0500B de Tektronix. Les différences sont spectaculaires. Dans le monde d'aujourd'hui, les signaux ont des contours plus rapides et des marges plus étroites, de sorte que le concepteur doit faire l'effort de modéliser, d'effectuer des simulations et de valider la conception. Lorsque vous consacrez autant de temps et d'efforts au processus de conception, vous ne voulez pas remettre en question le système de mesure ou passer plus de temps à résoudre les problèmes en raison de l'imprécision des mesures. Dans ce cas, on peut se demander si les effets de la charge de la sonde ne sont pas le maillon faible de la validation de la conception.
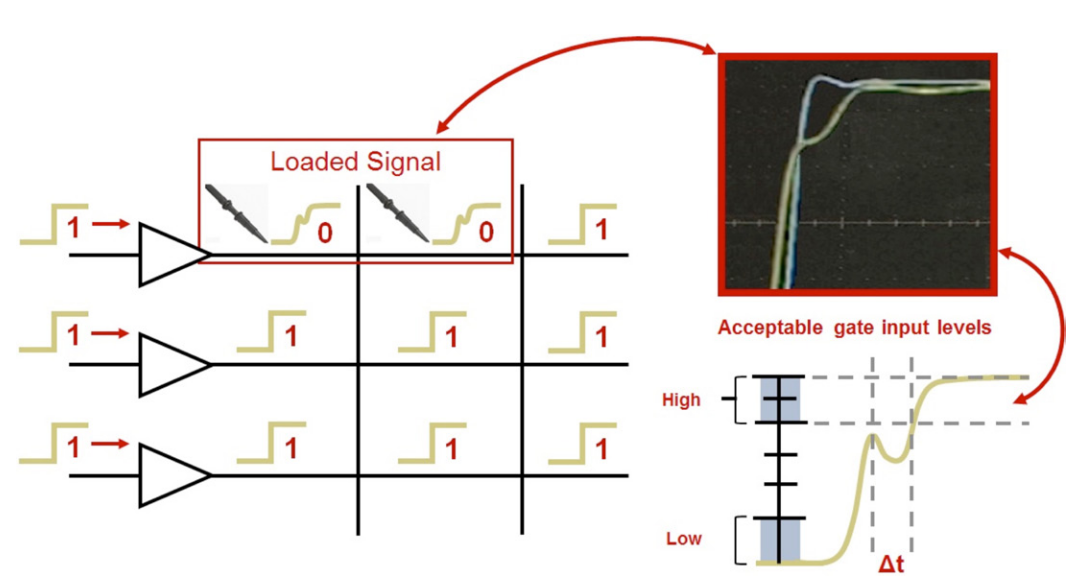
L'effet de charge d'une sonde peut non seulement entraîner une imprécision de la mesure, mais aussi affecter le fonctionnement du circuit. Dans l'exemple de la figure 10, la grande capacité d'entrée de la sonde a fait que le signal n'a pas respecté les exigences critiques en matière de temps d'établissement et de maintien. Vous pouvez également voir comment un bord avant dégradé ou un anneau peut provoquer des réflexions qui compromettent la fidélité du signal. Lorsque vous validez votre conception, la dernière chose que vous voulez faire est de vous battre avec l'équipement de test. Il est essentiel de sélectionner une sonde qui causera moins de perturbations en choisissant une sonde à faible capacité d'entrée.
Découvrez comment les sondes affectent les mesures
La vidéo montre une comparaison entre ce qui se passe au point de test et ce à quoi ressemble la sortie de la sonde Cliquez ici.
Conclusion
Lorsqu'ils choisissent une sonde, les utilisateurs s'intéressent d'abord à ce qui est considéré comme les spécifications de la bannière, à savoir la largeur de bande et la plage dynamique. Un utilisateur plus averti peut même vérifier la résistance d'entrée pour s'assurer qu'il s'agit d'une valeur importante. Cependant, une spécification critique souvent négligée est la capacité d'entrée de la sonde. Il est important d'utiliser une sonde dont la capacité d'entrée est plus faible, car elle causera moins de perturbations ou de charges.
Il se peut que vous utilisiez une sonde avec une capacité d'entrée plus importante et que vous obteniez les résultats souhaités. La question est de savoir si ces résultats sont réels et fiables. Il peut se passer plus de choses au point de test que vous ne le pensiez et ce n'est pas parce que la sonde reconstruit un signal que vous vous attendez à voir que vos résultats sont exacts. L'utilisation d'une sonde dotée d'une capacité d'entrée plus faible entraînera moins de perturbations ou de charges au point de test, ce qui augmente considérablement la probabilité d'obtenir une mesure plus précise.
Trouvez d’autres ressources précieuses sur TEK.COM
Copyright © Tektronix. Tous droits réservés. Les produits Tektronix sont protégés par des brevets américains et étrangers déjà déposés ou en cours d'obtention. Les informations contenues dans le présent document remplacent toutes celles publiées précédemment. Les spécifications et les prix peuvent être soumis à modification. TEKTRONIX et TEK sont des marques déposées de Tektronix, Inc. Toutes les autres marques de commerce, de services ou marques déposées sont la propriété de leurs détenteurs respectifs.
01/14 51W-30013-0

